
Imagine you’re running a 10K. Now imagine you’re running a 10K and you had a three-egg omelette, raisin toast, grits, and a side of hash browns for breakfast. You make it through the first 5 kilometers in 30 minutes and then your stomach starts to fight back. You decide it would probably be best for you (and certainly for any onlookers) if you cut your pace in half. That means in the next 30 minutes you run 2.5 kilometers. An hour into the race, a sharp pain in your side and some disconcerting bubbling tell you it’s time to slow down a little more, so you again cut your pace in half and go another 1.25 kilometers.
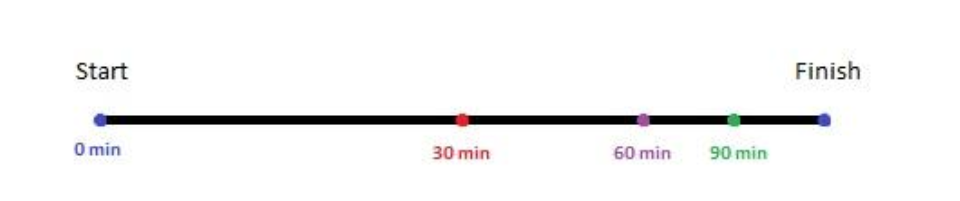
Every 30 minutes you cut your distance to the finish line in half, but you never get there.
After 90 minutes, the smart move is probably to quit the race and swear off anything but muesli for a few weeks. But imagine if you kept on with this pattern of running for 30 minutes, cutting your speed in half, running for another half hour, halving your speed again, etc. What would happen? At the end of each half hour run you’d be half as far from the finish line as you were at the beginning, but you would never get all the way there no matter how long you kept running.
That doesn’t completely answer the question “what would happen,” though. No, you will never actually reach the finish line, but you will converge to it. Intuitively “converge” seems like a good description, but what does it actually mean for you to converge to the finish line? As with any good math problem, it’s gonna take some trial and error to figure out. Let’s start by guessing at a possible definition of the sentence “you converge to the finish line” and modify it until it really captures the situation.
Guess 1: You are constantly moving toward the finish line.
There are two problems with this guess. Here’s the first one: Imagine there’s a greenhouse located two kilometers past the finish line. With each passing second you get closer to the greenhouse, but it would be wrong to say you’re converging to it. If you never even get close enough to smell the fertilizer, it doesn’t seem appropriate to say you’re converging to a greenhouse. Although each passing second brings you closer to both the finish line and the greenhouse, you only ever get close (we’ll have to define that better later on, but let’s just leave it as “close” for now) to the finish line. With the greenhouse in the distance, it’s safe to say that constantly moving towards a point isn’t enough to make you converge to it.
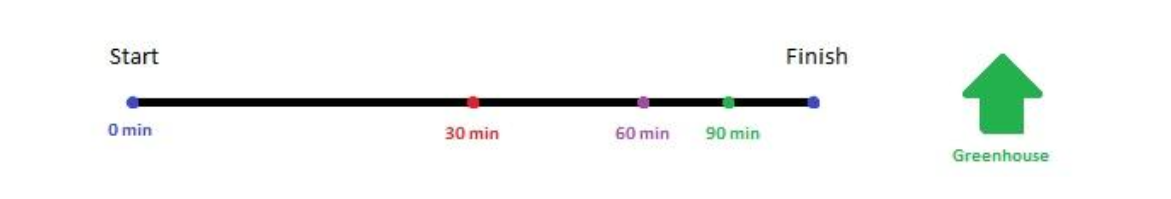
Although you are constantly getting closer to the greenhouse, you never even get within two kilometers of it.
Another reason Guess 1 is off is that we can imagine situations in which you converge to a point, but you’re not always moving closer to it. Imagine a ride where we strap you into a tire and push the tire down a halfpipe. Aside from a lot of screaming and a horrendous lawsuit, what do we expect to happen? First you’ll go down the left side and then up the right side, stopping partway up. Then you’ll switch directions and go down the right side and partway up the left side. However, when you stop and switch directions the second time, you’ll be a little bit lower than your first stop-and-switch (the one on the right side). This will continue with you going back and forth, stopping a little bit lower each time.
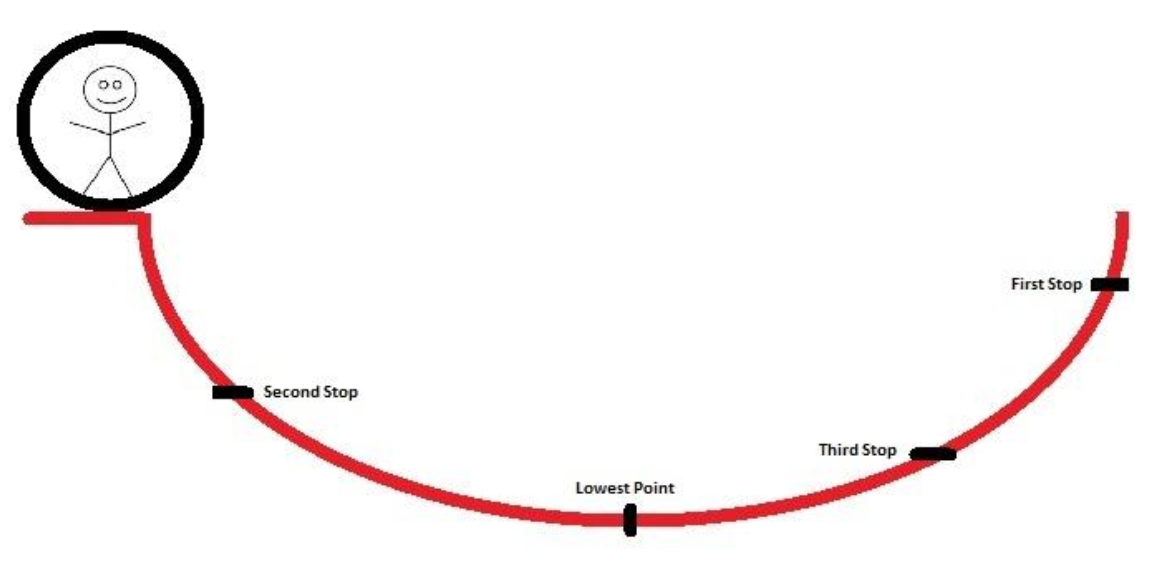
Fasten your seatbelts, it's going to be a bumpy night!
In our macroscopic real world experience, you’ll eventually come to rest at the lowest point on the halfpipe and stagger off the ride. But it’s not too hard to imagine an idealized setting, in which would just roll back and forth forever with the ‘stop-and-switch’s getting very close (again we’ll have to define that better later on) to the lowest point. Of course, you’re not going toward the lowest point for the whole ride. In fact, you will pass the lowest point infinitely many times and each time you pass it, you will overshoot and move away from it for a little while before turning back and heading towards it again. But even though each second isn’t carrying you closer to the lowest point, it does seem appropriate to say that you are converging to it. So what do our first example of converging to the finish line in the 10K and this example of converging to the lowest point in the halfpipe have in common? Just as importantly, what do they have in common that the example of running towards the greenhouse without ever getting close lacks? Now it’s time for our next guess at a definition of the sentence “you converge to the finish line.”
Guess 2: You get very close to the finish line.
Okay, if I put off defining “close” any longer, I’m going to start losing sleep. The conceptual challenge in this definition is coming to terms with imagining that we have infinite time. In our examples of convergence to the finish line and halfpipe base, we imagine continuing on forever. In the 10K, you go on forever halving your speed every 30 minutes, and on the halfpipe you go on forever rolling down one side, overshooting the lowest point, and rolling up a little lower on the other side.
To see what I mean by “close,” imagine my friend Adam and I are watching your 10K (and that we’ve got a lot of time on our hands). Once we get wise to your habit of cutting your speed in half every 30 minutes, we decide to pass the time by playing a little game. Adam says, “I bet you that she crashes before she gets within a kilometer of the end.”
I mull it over in my head and jot down some computations. Then I reply indignantly, “Like hell she won’t; that kid’s got the heart of a lion! Two hours into the race, she will have crossed the 9 kilometer mark.”
How did I figure this out? Each half hour you cut your speed in half and, as I mentioned before, go half as far as you did in the previous half hour. That means that in the first half hour you went 5 km, the next half hour you went 2.5 km, then 1.25 km, and then another 0.625 km. Putting this all together, after 4 half hour segments (i.e. two hours into the race) you will have travelled 5 + 2.5 + 1.25 + 0.625 km = 9.375 km, well within a kilometer of the end.
Humiliated in defeat, Adam comes right back at me. “Fine fine,” he says, “but there’s no way she makes it within a meter of the finish line.”
Eager to defend your honor, I get back to my computations. I beam triumphantly after a few minutes and say, “That kid could withstand a hurricane if she had to; she can sure as hell make it to that last meter. Mark my words; 7 hours into the race, she’ll be there.”
Again, how did I know? Well, after the first half hour, you made it halfway down the track and so you had half the track to go. In the following half hour, you made it halfway down the remaining stretch, meaning you had a quarter of the track left. After another half hour, you made it halfway down that remaining quarter -- leaving you with an eighth of the track ahead of you. If you look back at the first diagram (copied below) you’ll see what I mean.
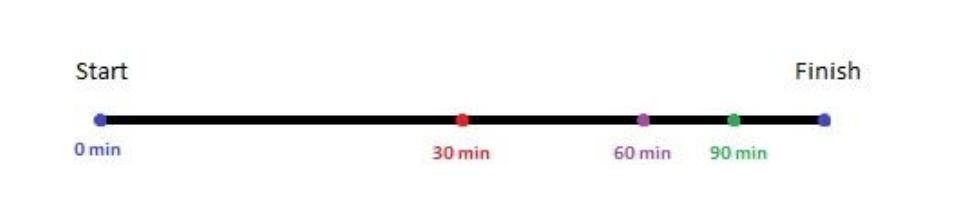
I don’t want to tire out your scrolling finger.
The upshot is that, with each half hour you divide your distance to the finish line by 2. Since the 10 kilometer track is 10,000 meters (Google “10 km in meters” if you don’t believe me), Adam was claiming that you would never cut down the distance ahead of you to one ten-thousandth of the original track. However, after 7 hours, you have cut the distance ahead of you in half 14 times (every 30 minutes) making it 1/16384 of the original track. Because 1/16384 of the original track is less than a meter, I get to laugh in Adam’s face.
Here’s the thing: No matter how small a distance Adam says, be it 1 km, 1 meter, or one gargoylillionth of a meter, given enough time, you will get there. It may take 500 years to get within one gargoylillionth of a meter, but (and here’s the infinity part) as long as we give you any amount of time you need, eventually you’ll get there.
I should mention two caveats to that last paragraph. The first is that in order to provide you with any amount of time you need, the three of us would have to be able to stay at the racetrack forever, which is unrealistic (see the HEALTH section of the Concepts Project for some explanations). The second caveat is that gargoylillion is a number I just made up so it would be a risky move to use it on a test.
The upshot is that when I say “you get very close to the finish line,” what I really mean is “you get arbitrarily close to the finish line.” In other words, for any arbitrary distance (greater than 0) that Adam names, eventually you will get that close to the finish line, as long as we make the weird and unrealistic assumption that you have forever to run. But, just in one day you would get within a trillionth of a meter of the finish line, which is probably good enough for any practical purpose. So when I say that you’re converging to the finish line, that can have some practical meaning. I’m sure the judges would call the race finished if they couldn’t see the distance between the edge of your shoe and the finish line.
After all that careful analysis, defining, and gambling, let’s evaluate our clarified Guess 2.
Guess 2, Clarified: You get arbitrarily close to the finish line.
This is almost what we want, but it ignores an important requirement. After all, in the first half hour of the race, you got arbitrarily close to the halfway point. In fact you did better than that; you actually got there. But it doesn’t make any sense to say you’re converging to the halfway mark. Actually, once you passed the halfway mark, you spent the rest of the race moving away from it. The upshot is that it’s not enough for you to get arbitrarily close; you have to get arbitrarily close and then stay close.
It wouldn’t do you a whole lot of good to get to the last meter of the race and then start running backwards and never make it to the last meter again. What we care about is that, after enough time has passed (7 hours in this case), not only are you within one meter of the finish line, but you will forever stay within one meter.
This is a little more interesting in the halfpipe example. If you reach the lowest point after 2 seconds, we can say you got within a centimeter of the lowest point in 2 seconds. But after another second, you might be half way up the right side so you didn’t stay within a centimeter of the lowest point. Over and over you will get within a centimeter of the lowest point and then wind up more than a centimeter away from it on the other side. However, after a long enough time (assuming you keep rolling forever), although you’ll still be going back and forth past the lowest point, you’ll never overshoot by more than a centimeter. Then, even though it isn’t your first time getting close, from that point on you will stay close.
In terms of my game with Adam, here’s what we mean when we say that “you converge to the finish line:” If Adam challenges you to get within any distance (greater than 0) of the finish line, no matter how small the challenge distance is, I can name some time after which you will never be further than it from the finish line. In other words, for any arbitrary distance (greater than zero), after enough time you will never be further than that or, more pithily but precisely…
Answer: You get arbitrarily close and you stay that close.
Even though you never make it to the finish line, I’d say converging to it is almost as good. Still, next time I’d suggest a lighter breakfast.
There are many examples in everyday life of quantities converging over time; here's one from probability. Imagine you go into a casino with a perfectly fair roulette wheel, i.e. it’s divided into 36 equally sized slots, 18 of which are red and the other 18 of which are black. Of course in practice there’s a trick that gives the casino an advantage, but we’re going to imagine you’ve stumbled into the most just and equitable casino on Las Vegas Blvd. If you bet on red 10 spins in a row, you “expect” to win half the spins, but in practice you are almost as likely to win 4 as you are to win 5.
With the language of convergence, we are better equipped to conceive of “expecting” to win half the spins. What we really mean is that if you keep on spinning over and over and over, as time goes on, the fraction of the spins that you win will converge to 50%. It’s an elegant mental picture: The fair game doesn’t mean that you will win exactly half the time. But if you keep playing, no matter how close to 50% you want your win percentage to get, eventually it will get that close and stay that close. That said, it may take a very long time for the numbers to balance out so you probably want to think twice before you put it all on red.
